Option Greeks are tricky beasts with their complex formulae and intimidating names. This article is not about the formulae at all. The idea is to discuss what each of the Greeks represent, and understand what drives each of them. Often the PRMIA exam will ask a question about a Greek, and very likely that question will expect you to understand the relationship between the variable and the underlying asset, and how the Greeks can be used to measure and manage risks.
The ‘Greeks’ measure the sensitivity of an option’s value to its underlying parameters, ie price of the underlying, interest rate, passage of time etc. Without further introduction, let us launch in and see what they are. Your text book will have a great deal of detail on each of the Greeks. They also have rather daunting formulae, but it is unlikely that you will be asked to calculate the Greeks except in an intuitive way (eg, using the put-call parity).
The PRMIA candidate’s focus should be less on the formulae and more on an intuitive understanding of these variables, how they behave in response to different changes, and what their relationship is with each other. One way to learn about these is to experiment and see how the values of the Greeks changes as different option valuation inputs are changed.
You can use the spreadsheet here that has the formulae for the various Greeks built in to play around and discover.
Delta
Delta is the sensitivity of an option’s price to changes in the value of the underlying. It is equal to:
Changes in option value/Change in the price of the underlying
Practically, delta is the change in option value for every $1 change in the price of the underlying. Note that:
- Positive delta means that both the option value and the underlying move in the same direction
- Negative delta means that the option value and the underlying move in opposite directions.
- Long calls have positive delta, and long puts have negative delta. By extension, short calls have negative delta, and short puts have positive delta.
For a stock, our proxy in this discussion here for the underlying, delta will be exactly one. A long stock position has a delta of +1, and a short stock position has a delta of -1. Options contracts come in packages of 100 shares, so a per share delta of 0.13 may be expressed as a delta of 13 for the contract.
Delta is additive
An important thing to remember about delta (and in fact all the Greeks) is that it is additive when there are multiple securities in a portfolio that carry delta or any other risk expressed by a Greek.
Because a Long Call + Short Put = Long stock,
ðCall Delta + Delta of a Short Put = Stock Delta
Or, Call Delta – Put Delta = Stock Delta (∵ Delta of a short put = – delta of a long put)
We know that the RHS = 1. So if we know the delta of either the call or the put, we can calculate the delta of the other. The thing that can be confusing here is that an exam question may ask the delta of a put, or may provide the delta of the put, which means the delta of a long put position. The delta of the short put position would be the negative of that number. An easier way to remember this may be that the sum of the absolute values of the deltas of a call and a put is equal to 1.
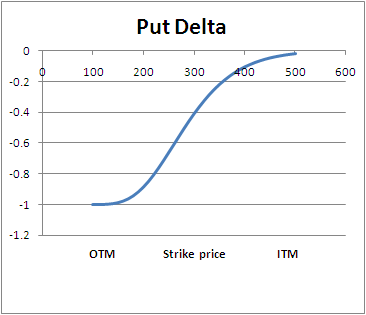
Call deltas can be interpreted as the probability of the option finishing in-the-money. For this reason, a deep in-the-money option will have a delta close to 1, and a deep out-of-the-money option will have a delta close to 0.
The following graphs demonstrate how call and put deltas behave as the price of the underlying changes. These reflect long positions, and it should be easy to visualize the behavior of the delta of short positions too using these graphs.
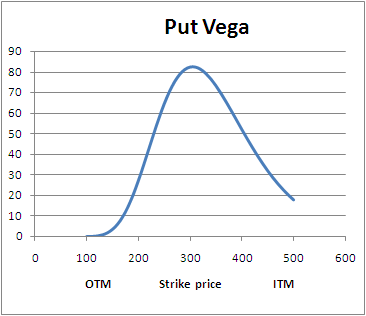
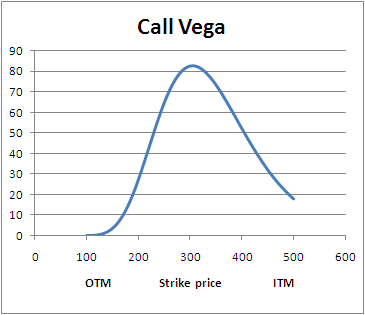
All graphs below use a strike price of 300.
Delta as the price of the underlying changes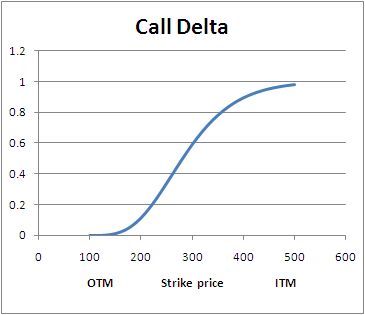
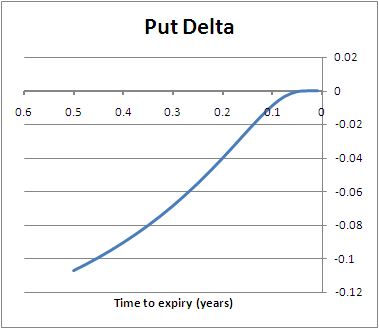
Delta and time to expiry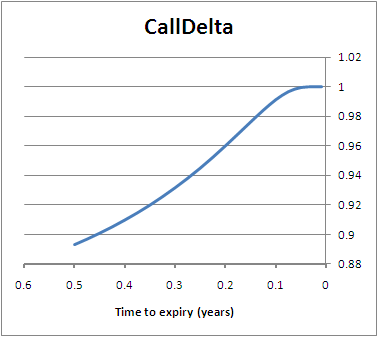
Gamma
If delta is like a first derivative of the option value with respect to the price, gamma is the second derivative. It measures the rate of change in the delta as a result of changes in the price of the underlying.
Ie, Gamma = Change in delta/Change in price of the underlying
A positive gamma means delta increases with an increase in price, and negative gamma means the opposite. A gamma of 0.08 and a delta of 0.50 means a 1 point positive change in the value of the underlying would increase the delta to 0.58. Again, since options trade in lots of 100, the gamma here would be referred to as 8 and not 0.08.
Remember the following:
· Long call, long puts: Have positive gamma
· Short call, short puts: Have negative gamma
· Pure stock positions: Have no gamma, ie zero gamma.
For a deep in-the-money option, delta approaches 1 and gamma approaches zero. Similarly delta for far out-of-the-money options will be close to zero as delta is already zero.
Calls and puts have different deltas (recall that the sum of their absolute values is 1). However, they have the same gamma for the same option. This is intuitive if you think about the put-call parity: Call Delta – Put Delta = 1, therefore gamma has to be the same for both otherwise one will change more than the other and this identity will not be maintained.
Since gamma also follows the put-call parity, Call Gamma – Put Gamma = Stock Gamma. Now the stock has a linear payoff, and gamma is zero as the second derivative is zero. Thus call gamma and put gamma will be identical.
Gamma is maximum at or near the exercise price, ie it is maximum when the option is at-the-money.
Theta
Theta measures time decay. An option’s value can be understood to comprise intrinsic value (ie, stock price minus exercise price), and extrinsic value (ie the time value of the option). Extrinsic value is driven by the fact that the value of the underlying is volatile, and the greater the time to expiry the greater the possibilities for the ultimate stock price and therefore greater the risk, justifying a higher value.
As an option gets closer to expiration, extrinsic value diminishes and only intrinsic value tends to remain. At expiration, intrinsic value is the only valuable thing left in the option.
Be careful about whether theta is daily or annual – generally option valuation formulae give an annual theta number which needs to be divided by the number of days in the year to get a daily value. Theta is a measure of the amount lost per time period (a day, a year), all other things remaining constant. By convention, thetas are negative as they indicate a loss of value. So a theta of 0.06/day means 6 cents are lost in value with the passage of each day.
Remember:
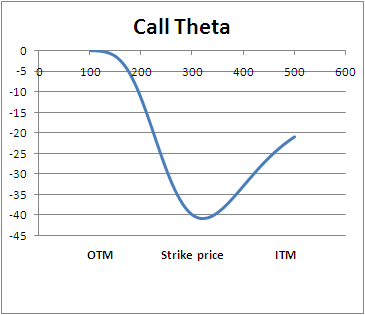
· At-the-money options have the greatest theta
· Theta is not linear, ie, option prices do not decline at a constant rate, but accelerate closer to expiration.
· The more in-the-money and out-of-the-money an option is, the more linear the theta graph gets
Theta would be positive for option sellers as they would benefit from the passage of time and the decline in the value of the option for which they have already received a premium. Remember that a negative theta means that you lose money with the passage of time if the underlying or other factors affecting the option price do not move.
Rho
Rho measures the sensitivity of option prices to changes in interest rates. Normally, the effect of rho pales in comparison to delta or theta. However, for very long term options such as LEAPS, rho can be a significant source of risk. It measures the change in option value from a 1% change in interest rates. Rho is positive for calls, and negative for puts. This is intuitive as call values will go up as the interest rates go up (as a call is the equivalent buying stock on borrowed money, and the interest which is a part of the option value goes up as a result of the increase in rates).
It is negative for puts for exactly the opposite reason (as a put is equivalent to selling stock short without receiving the proceeds, and with rising interest rates you can earn more interest on lent money outside, thus making the put less attractive).
Vega
Vega is the change in value from a 1% change in volatility. Vega is higher the further away we are from the exercise date. Vega is zero for the underlying, for any changes in volatility do not affect the price.